box muller normal distribution The Box–Muller transform, by George Edward Pelham Box and Mervin Edgar Muller, is a random number sampling method for generating pairs of independent, standard, normally distributed (zero expectation, unit variance) random numbers, given a source of uniformly distributed random numbers. The . See more Learn the art and science of welding and metal fabrication in a true shop environment with an emphasis on off-road and automotive applications. Benefit from an accelerated curriculum of welding and metal fabrication geared towards general manufacturing.
0 · quant girl box muller
1 · normal distribution of x 1
2 · how to simulate normal distributions
3 · c normal distribution generator
4 · box muller wikipedia
5 · box muller transformation
6 · box muller generator
Scope of Work: Fabrication is a comprehensive process encompassing the entire creation of metal structures or products, including cutting, shaping, bending, assembly, and finishing. Welding, on the other hand, is a specific process within fabrication focused solely on joining metal pieces together.
quant girl box muller
The Box–Muller transform, by George Edward Pelham Box and Mervin Edgar Muller, is a random number sampling method for generating pairs of independent, standard, normally distributed (zero expectation, unit variance) random numbers, given a source of uniformly distributed random numbers. The . See moreSuppose U1 and U2 are independent samples chosen from the uniform distribution on the unit interval (0, 1). Let See more
normal distribution of x 1
The polar method differs from the basic method in that it is a type of rejection sampling. It discards some generated random numbers, but can be faster than the basic method . See more
• Inverse transform sampling• Marsaglia polar method, similar transform to Box–Muller, which uses Cartesian coordinates, instead of polar coordinates See more
• Weisstein, Eric W. "Box-Muller Transformation". MathWorld.• How to Convert a Uniform Distribution to a Gaussian Distribution (C Code) See more
The polar form was first proposed by J. Bell and then modified by R. Knop. While several different versions of the polar method have been described, the version of R. Knop will be . See more
C++The standard Box–Muller transform generates values from the standard normal distribution (i.e. standard normal deviates) with mean 0 and standard deviation 1. The implementation below in standard See more A transformation which transforms from a two-dimensional continuous uniform distribution to a two-dimensional bivariate normal distribution (or complex normal distribution).
There are many methods to generate Gaussian-distributed numbers from a regular RNG. The Box-Muller transform is commonly used. It correctly produces values with a normal distribution. The math is easy. You .
how to simulate normal distributions
The Box–Muller transform is a pseudo-random number sampling method for generating pairs of independent, standard, normally distributed (zero expectation, unit variance) random numbers, given a source of uniformly . Exercise (Box–Muller method): Let U and V be independent random variables that are uniformly distributed on [0, 1]. Define X: = √− 2log(U)cos(2πV) and Y: = √− .A Box Muller transform takes a continuous, two dimensional uniform distribution and transforms it to a normal distribution. It is widely used in statistical sampling, and is an easy to run, elegant .The Box Muller method is a brilliant trick to overcome this by producing two independent standard normals from two independent uniforms. It is based on the familiar trick for calculating. I = .
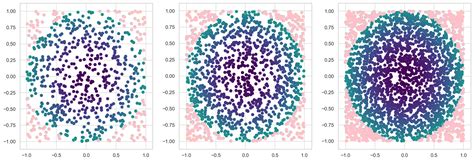
Here’s the Box-Muller method for simulating two (independent) standard normal variables with two (independent) uniform random variables. Two (independent) standard . Sampling using the Box-Muller Transform¶ The Box-Muller transform is a neat little "trick" that allows us to sample from a pair of normally distributed variables using a source of . Box-Muller transform is a method used to produce a normal distribution. Imagine two independent distributions of X, Y ~N(0,1) plotted in the Cartesian field.
The Box–Muller transform, by George Edward Pelham Box and Mervin Edgar Muller, [1] is a random number sampling method for generating pairs of independent, standard, normally distributed (zero expectation, unit variance) random numbers, given a source of uniformly distributed random numbers.
A transformation which transforms from a two-dimensional continuous uniform distribution to a two-dimensional bivariate normal distribution (or complex normal distribution).
There are many methods to generate Gaussian-distributed numbers from a regular RNG. The Box-Muller transform is commonly used. It correctly produces values with a normal distribution. The math is easy. You generate two (uniform) random numbers, and by applying an formula to them, you get two normally distributed random numbers. The Box–Muller transform is a pseudo-random number sampling method for generating pairs of independent, standard, normally distributed (zero expectation, unit variance) random numbers, given a source of uniformly distributed random numbers. Exercise (Box–Muller method): Let U and V be independent random variables that are uniformly distributed on [0, 1]. Define X: = √− 2log(U)cos(2πV) and Y: = √− 2log(U)sin(2πV). Show that X and Y are independent and N0, 1 -distributed. How can I convert a uniform distribution (as most random number generators produce, e.g. between 0.0 and 1.0) into a normal distribution? What if I want a mean and standard deviation of my choosing?
A Box Muller transform takes a continuous, two dimensional uniform distribution and transforms it to a normal distribution. It is widely used in statistical sampling, and is an easy to run, elegant way to come up with a standard normal model .The Box Muller method is a brilliant trick to overcome this by producing two independent standard normals from two independent uniforms. It is based on the familiar trick for calculating. I = e−x2/2dx .
Here’s the Box-Muller method for simulating two (independent) standard normal variables with two (independent) uniform random variables. Two (independent) standard normal random variable Z1 and Z2. Generate two (independent) uniform random variables U1 ∼ U(0, 1) and U2 ∼ U(0, 1). Sampling using the Box-Muller Transform¶ The Box-Muller transform is a neat little "trick" that allows us to sample from a pair of normally distributed variables using a source of only uniformly distributed variables. The transform is actually pretty simple to compute.The Box–Muller transform, by George Edward Pelham Box and Mervin Edgar Muller, [1] is a random number sampling method for generating pairs of independent, standard, normally distributed (zero expectation, unit variance) random numbers, given a source of uniformly distributed random numbers. A transformation which transforms from a two-dimensional continuous uniform distribution to a two-dimensional bivariate normal distribution (or complex normal distribution).
cnc machine for sale bc canada
There are many methods to generate Gaussian-distributed numbers from a regular RNG. The Box-Muller transform is commonly used. It correctly produces values with a normal distribution. The math is easy. You generate two (uniform) random numbers, and by applying an formula to them, you get two normally distributed random numbers.

The Box–Muller transform is a pseudo-random number sampling method for generating pairs of independent, standard, normally distributed (zero expectation, unit variance) random numbers, given a source of uniformly distributed random numbers. Exercise (Box–Muller method): Let U and V be independent random variables that are uniformly distributed on [0, 1]. Define X: = √− 2log(U)cos(2πV) and Y: = √− 2log(U)sin(2πV). Show that X and Y are independent and N0, 1 -distributed.
How can I convert a uniform distribution (as most random number generators produce, e.g. between 0.0 and 1.0) into a normal distribution? What if I want a mean and standard deviation of my choosing?
c normal distribution generator
A Box Muller transform takes a continuous, two dimensional uniform distribution and transforms it to a normal distribution. It is widely used in statistical sampling, and is an easy to run, elegant way to come up with a standard normal model .The Box Muller method is a brilliant trick to overcome this by producing two independent standard normals from two independent uniforms. It is based on the familiar trick for calculating. I = e−x2/2dx .
box muller wikipedia
Here’s the Box-Muller method for simulating two (independent) standard normal variables with two (independent) uniform random variables. Two (independent) standard normal random variable Z1 and Z2. Generate two (independent) uniform random variables U1 ∼ U(0, 1) and U2 ∼ U(0, 1).
box muller transformation
"Keep your workshop or garage organized with a Stack-On, two-shelf wall cabinet. This welded steel cabinet comes mostly assembled. The door handles can be mounted in minutes. Cabinet has two fixed shelves that can hold up to 65 lbs. each. Doors close with double enforced powerful magnetic strip that holds the cabinet door securely closed.
box muller normal distribution|normal distribution of x 1